Snyder-Stavola model

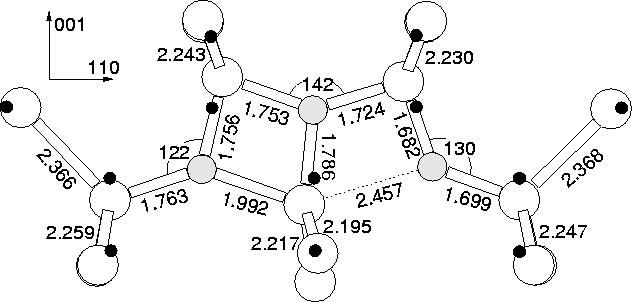
The relaxed structure is shown in Figure 9.13 with eigenvalues in Figure 9.10a. When unconstrained, the central Si atom moves $\sim$0.05 Å off the C₂ axis to form a structure somewhere between the Snyder-Stavola and an oxygen square with a flanking O_i, saving 0.063 eV in the process. This is consistent with calculations by Chadi who found an energy saving of 0.07 eV for the same process[211]. This is presumably the diffusion barrier for reorientation of the defect between the two off-site configurations, and hence it would appear time averaged C_2v.

The vibrational modes of the Snyder-Stavola structure are given in Table 9.5. The high frequency mode is due to out-of-plane wag of the core trivalent oxygen atom, and seems remarkably high for such a motion. The modes are not in good agreement with either TD1/TD2, which exhibit two modes each at 975/988 and 716/724 cm^-1. The only possibility for correlation is if the calculated modes at 978.9 and 771.6 cm^-1 are those that have been observed, and there are additional experimental modes in the 850-900 cm^-1 range which have not yet been observed. The TD2 988 cm^-1 mode drops by 43 cm^-1 when ¹⁶O is replaced with ¹⁸O, which is not very good agreement with the 978.9 cm^-1 mode shift of 46.9 cm^-1.

We therefore conclude on the basis of the vibrational modes that the Snyder-Stavola model is unlikely to be TD2, but may be TD1 if there are further vibrational modes which have not yet been detected by experiment.

 

3cLocal Vibrational Modes (cm^-1) Dipole moment squared

¹⁶O ¹⁷O ¹⁸O for ¹⁶ O

1230.2 26.2 49.8 0.421

1038.5 25.2 48.2 0.031

978.9 24.6 46.9 0.140

946.2 26.0 49.6 0.005

899.2 20.6 39.3 0.120

884.6 22.3 42.7 0.143

771.6 17.4 32.8 0.190

755.6 17.8 33.8 0.117

697.5 13.4 24.5 0.034

630.8 1.8 3.8 0.005

601.2 2.9 6.4 0.014

560.7 0.9 1.9 0.001

538.7 0.3 0.6 0.020

527.6 0.2 0.5 0.001

  

Figure 9.13: The core of the Snyder/Stavola 3O thermal donor model (lengths in Å). Black dots mark ideal Si lattice sites, showing the [001] compression and [110] lattice tension. The deviation from C_2v symmetry saves 0.063 eV over the relaxed C_2v symmetric structure.