Spin averaged $\tilde{E}_{xc}$ and improvements to the approximations

In the spin averaged case,

$$\tilde{E}_{xc} = \sum_k d_k \int h_k({\bf r}) \varepsilon_{xc}(\tilde{n}) d{\bf r}.$$ (26)

If h_k is a Gaussian then the integrals are proportional to $\langle \varepsilon_{xc}(\tilde{n})\rangle_k$. To a first approximation, since $\varepsilon_{xc}(n)$ only varies slowly with n we can approximate this to $\varepsilon_{xc}(\langle\tilde{n}\rangle_k)$, where

This approximation can be improved through some mathematical tricks, since we know that a highly accurate form for $\varepsilon_{xc}(n)$ is given by $\varepsilon_{xc}(n) = An^s$ where s=0.30917 (see Eq. 2.5.19).

If we define

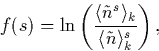$$f(s) = \ln\left(\frac{\langle\tilde{n}^s\rangle_k}{\langle\tilde{n}\rangle^s_k}\right),$$ (27)

we can now do a limited expansion of this to give an approximation for f(s),

$$f(s) = \frac{1}{2}s(s-1)f(2).$$ (28)

This step is one of the largest approximations within our method, leading to errors of up to 10%; however in practise its contribution to the total energy is minute and so the approximation is not unreasonable.

To find f(2) we need to know the second moment of $\tilde{n}$, which can be determined analytically as

 

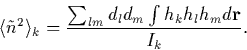$$\langle \tilde{n}^2 \rangle_k = \frac{ \sum_{lm}d_ld_m \int h_k h_l h_m d {\bf r}}{I_k}.$$ (29)

Finally substituting all of these new terms back in, we get an expression for $\tilde{E}_{xc}$: