Hydrogenated Vacancy Centres

  Schematic diagrams of the hydrogenated vacancy centres are shown in Figure 4.3. The top Kohn-Sham eigenvalues of the hydrogenated vacancies are shown in Figure 4.4. These show that $\rm V_{In}H_4$ is a single shallow donor. Each of the defects considered has a single shallow level close to the conduction band. We chose cluster charge states to empty this level, since in practise it would thermally depopulate. $\rm V_{In}H_4$ contains one electron in this level when neutral and hence is a single shallow donor. All of the defects also possess a t₂-like state above the top of the valence band which splits as the number of H atoms decreases.

  

Figure 4.3: Schematic diagrams showing $\rm V_{In}H_n, n=4,0$. The tetrahedral symmetry is shaded in $\rm V_{In}H_4$.

  

Figure 4.4: Top Kohn-Sham eigenvalues of the hydrogenated vacancies, (a) $\rm V_{In}H_4^+$, (b) $\rm V_{In}H_3$, (c) $\rm V_{In}H_2^-$, (d) $\rm V_{In}H^{2-}$, (e) $\rm V_{In}^{3-}$. Filled boxes indicate electrons and empty boxes indicate holes. The eigenvalues have been arbitrarily shifted to align the highest filled level below the t₂-like state with zero.

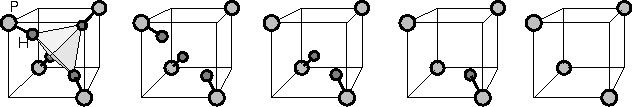
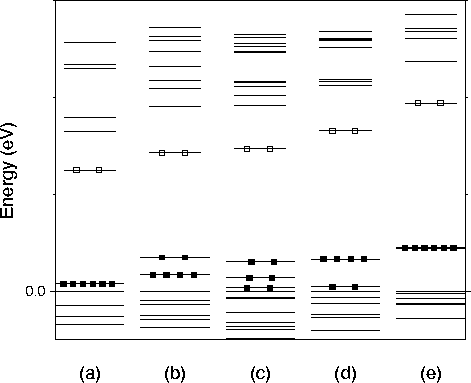As each hydrogen atom is removed from the vacancy, an electron is removed from the highest occupied state. The resulting defects can act as acceptors that fill the t₂ -level when ionised. This level is already filled in the case of $\rm V_{In}H_3$ so this defect is electrically neutral. However $\rm V_{In}H_2$, $\rm V_{In}H$ and $\rm V_{In}$ will behave as single, double and triple acceptors respectively.

The t₂-level is split due to the lower symmetry of the partially hydrogenated vacancies. $\rm V_{In}H_4^+$ has T_d symmetry and therefore the level does not split. $\rm V_{In}H_3$ has C_3v symmetry, so the t₂ splits into an e- and a₁- level. $\rm V_{In}H_2^-$ has C_2v symmetry which leads to three separated singlets. $\rm V_{In}H^{2-}$ and $\rm V_{In}^{3-}$ are directly comparable with $\rm V_{In}H_3$ and $\rm V_{In}H_4^+$, with the dangling and hydrogen terminated bonds reversed, in addition to the a - e level ordering. This t₂-like state gradually moves upwards away from the valence band as hydrogen atoms are removed. In $\rm V_{In}^{3-}$ the t₂-level is quite deep in the gap.

The indium vacancy, $\rm V_{In}^{3-}$, fits the trend of increasing acceptor character with decreasing number of hydrogen atoms present. Previous calculations [68] also show $\rm V_{In}$ to be a triple acceptor.

The local vibrational modes for $\rm V_{In}H_4$ with pure and mixed isotope composition are shown in Table 4.3. The highest IR visible mode for $\rm V_{In}H_4^+$ at 2356.4 cm^-1 is in excellent agreement with experiment (2315.6 cm^-1, a 1.8% error), as is the 1690.8 cm^-1 mode for $\rm V_{In}D_4^+$ (experimental value of 1683.4 cm^-1, an error of 0.4%). This small drop in error with deuteration suggests only limited anharmonic character in the bonding [37].

 

H₄ H₃D H₂D₂ HD₃ D₄

* 2387.85 2380.18 2372.34 2364.31 * 1713.01

T 2356.40 D 2356.32 2356.24 1707.26 T 1690.82

D 618.57 1696.43 1701.74 D 1690.95 D 445.34

T 565.87 D 603.12 1691.08 D 549.65 T 411.94

T 408.69 565.71 595.02 D 428.92

D 508.72 556.04 412.03

408.69 541.80 481.28 425.83

The LVMs for the range of hydrogenated vacancies, $\rm V_{In}H_n, n = 0,4$ are shown in Table 4.4. The results show that as the number of H atoms in the vacancy increases, the P-H bonds are shorten and the vibrational modes increase. This is due to the compressive effect of the other hydrogen atoms on each P-H group, coupled with the removal of dangling bonds from `unsaturated' P atoms which would have acted to attract the hydrogen away from its phosphorus neighbour. This is consistent with previous results obtained for Si [60,69].

 

Defect 5cLocal Vibrational Modes Symmetry Bond

Exp [61] [59] ([70]) 3cCalculation Length

$$\rm V_{In}H_4^+$$ 2315.2 2315.6 2387.8* 2356.4T 618.6D T_d 1.419

$$\rm V_{In}D_4^+$$ 1683.4 1713.0* 1690.8T 445.3D

$$\rm V_{In}H_3$$ 2324.1 2286.3D 695.3D C_3v 1.429

$$\rm V_{In}D_3$$ 1667.6 1640.8D 498.2D

$$\rm V_{In}H_2^-$$ 2256.2 2216.8 730.0 C_2v 1.439

617.7 611.8 602.0

$$\rm V_{In}D_2^-$$ 1619.4 1591.6 523.0

446.4 444.4 438.8

$$\rm V_{In}H^{2-}$$ 2201.7 (2202.4) 2150.7 644.9 644.7 C_3v 1.450

$$\rm V_{In}D^{2-}$$ 1603.8 1544.7 467.5 467.4

The shift in P-H length from $\rm V_{In}H_4$ to $\rm V_{In}H$ is only 2.1% (from 1.419 Å  to 1.450 Å), but it leads to a 9.9% shift in vibrational mode (2387.8 cm^-1 to 2150.7 cm^-1). The calculated H- stretch modes of the partially hydrogenated vacancies could account for a group of experimentally observed vibrational modes lying between 2200 and 2290 cm^-1 [59,70].

High temperature annealing of InP:Fe reduces the concentration of $\rm V_{In}H_4^+$. This is due to the partial dissociation of this centre and, up until now, only $\rm V_{In}H$ has been identified with certainty. It is shown here that $\rm V_{In}H_2$ and $\rm V_{In}H$ are acceptors. In annealed high-resistivity material they should cause a drop in the $\rm Fe^{2+}$ concentration. This will be in addition to the decrease in concentration of $\rm Fe^{2+}$ due to loss of the $\rm V_{In}H_4$ donor.

In contrast, Bardeleben et al [71] found that thermal annealing of Fe doped InP in the range 660-820 $^\circ$C led to an increase in $\rm Fe^{2+}$ concentration. They suggested this was due to the formation of some unidentified deep thermal donors. This could be associated with other hydrogen complexes. However, from this work it seems unlikely that hydrogenated vacancies are responsible.

In summary, the fully hydrogenated vacancy, $\rm V_{In}H_4$, acts as a single donor due to a partially filled singlet near the top of the gap; thus $\rm V_{In}H_4$ will compensate $\rm Fe^{3+}_{In}$ in InP. Removal of hydrogen atoms from the vacancy leads to increased acceptor character as the triplet state starts to empty.