Expanding the Wavefunction

The wavefunctions are expanded using a basis of localised orbitals, $\phi_i({\bf r} - {\bf R}_i)$, where

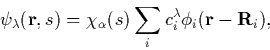$$\psi_\lambda({\bf r},s) = \chi_\alpha(s)\sum_i{c_i^\lambda\phi_i({\bf r} - {\bf R}_i)},$$ (17)

which converts the Kohn-Sham equations into matrix equations for $c_i^\lambda$. A set of Gaussian functions are used, multiplied by spherical functions to set the orbital quantum number:

 

$$\phi_i({\bf r} - {\bf R}_i) = (x - R_{ix})^{n_1}(y - R_{iy})^{n_2}(z - R_{iz})^{n_3}e^{-a_i({\bf r} - {\bf R}_i)^2}.$$ (18)

The choice of n₁, n₂ and n₃ in Eq. 3.1.2 sets the orbital type; n₁=n₂=n₃=0 gives spherically symmetric s-orbitals, setting one of n₁, n₂ or n₃ = 1 gives p-orbitals in the x, y or z direction respectively, whereas setting $\sum_i{n_i} = 2$ produces a combination of five d- and one s- type orbital.

The charge density for a given spin state can then be described in terms of the density matrix, b_ij,s (the total charge density is simply the sum of the spin dependent charge densities):

Here, s gives the spin state and $\lambda$ is an occupied orbital. Substituting this expression for n(r) back into Equation 2.4.14 is only simple for the kinetic and pseudopotential energy terms:

However the Hartree energy requires O(N⁴) integral terms, and when N, the number of basis functions is large, this rapidly becomes unfeasible. Equally the exchange-correlation energy needs some sort of simplification. We therefore introduce an approximate charge density for each spin, $\tilde{n}_s$, which allows analytic solution of these energies and the forces acting on each atom.