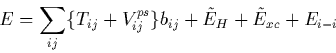
I will now attempt to summarise the method shown so far, and draw out the salient calculational points. Using the approximate functions for EH and Exc derived in the last few sections we can rewrite the total energy as
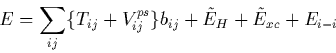 |
(31) |
where
E is now minimised, however an additional constraint has to be applied in order to keep the wavefunctions orthonormal; this can be imposed in terms of the overlap matrix, S,
Now all we need is Lagrange undetermined multipliers, ![]() to
allow unconstrained minimisation with respect to
to
allow unconstrained minimisation with respect to ![]() , i.e.:
, i.e.:
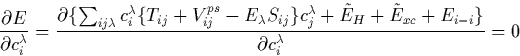 |
(32) |
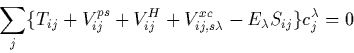 |
(33) |
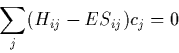 |
(34) |
Equation 3.2.39 is the Kohn-Sham equations once more, and is written in terms of two generalised eigenvalue problems (one for each spin).